We now get into the meat of the empirical aspects of plasmas. Our theoretical tools are wonderful but provide models that fall apart easily with a slight disturbance. Yet we do find repetitive processes at work and causes that can be tracked as well. All good but straining on our computer power to model.
In the meantime empirical knowledge is intuitive and surprisingly stable in its own right. Thus we have advanced steadily.
There just too many active centers in a plasma to allow predictive methods to ever be either exact or sufficiently inexact as well. It is a little like building large stone structures where you over engineer it all.
.
Essential Guide to the EU – Chapter 6 Currents, Filaments and Pinches
Planetary nebulas often exhibit characteristic bi-polar symmetries with a centered plasma pinch, polar jets, and an equatorial torus. Image courtesy NASA, ESA and the Hubble Heritage Team
https://www.thunderbolts.info/wp/2011/12/06/essential-guide-to-the-eu-chapter-6/
6.1 Thermal Motion and Current
It is important to distinguish between
random thermal motion and uniform linear motion in a plasma. The latter
is an electric current which flows due to the presence of an electric
field.
The random thermal motion is measured by
the temperature of the plasma, or by the temperatures of the ions and
electrons separately if their temperatures are different. This motion,
being a motion of charged particles, is also a form of current, but one
which oscillates about an average position, as opposed to moving in one
direction only. Strictly speaking, temperature can only be an accurate
measure of the energy if the distribution of velocities of individual
particles is Maxwellian, that is, if the distribution is equivalent to
that which would result from elastic collisions between the particles.
Uniform linear motion results from an electric field and represents a drift current. All particles with the same sign charge
(positive or negative) move in the same direction under the influence
of the electric field. That is, in a plasma, where there are roughly
equal numbers of positive and negative particles (“net neutrality”), we
find the positively charged particles moving together in a direction
opposite that of the electrons’ motion.
The particles all have kinetic energy,
which may be high, but they do not have a temperature as a result of
this linear motion. That’s because temperature is only used to measure
the energy of particles with random velocities, undergoing
collisions. As both types tend to move along more or less aligned or
parallel trajectories, and plasma densities are relatively low,
collisions are less common and Maxwellian collision conditions are not
obtained.
Both types of motion exist
simultaneously wherever a current is flowing. The current motion or
drift of the particles is superimposed on the random motions. Another
way of looking at this is to think of the mean position of the random
motion as moving with the drift velocity in the direction of the
current.
6.2 Electron and Ion Currents
We have seen that the electrons acquire
much higher velocities than ions due to their smaller mass.
However, an electron carries the same magnitude of (negative) charge as a positively charged proton, the lightest form of ion. Therefore, the higher velocity of the electrons means that they are more effective than the ions at carrying current in a plasma.
However, an electron carries the same magnitude of (negative) charge as a positively charged proton, the lightest form of ion. Therefore, the higher velocity of the electrons means that they are more effective than the ions at carrying current in a plasma.
The ratio of the electron current to the
ion current in a non-relativistic plasma current is proportional to the
square root of the inverse ratio of the masses. For the lightest
positive ion, a proton, this means that the electron current is around
43 times greater than the ion current. [ If the mass of an electron is
taken as 1, the mass of a proton would be about 1836 times greater):
√(1836 ÷ 1) = 42.85 ] In many situations, it is the motion of the
electrons which determines the plasma behavior.
6.3 Current in Laboratory Discharge Tubes
Plasma has been studied in laboratory
experiments for over a hundred years, and a vast amount of experimental
data and analysis is now available. One of the fundamental experiments
involves a Glow Discharge Tube in which a current is passed through a
low-pressure gas such as mercury vapor. This causes ionization of the
gas and creation of a plasma within the tube.
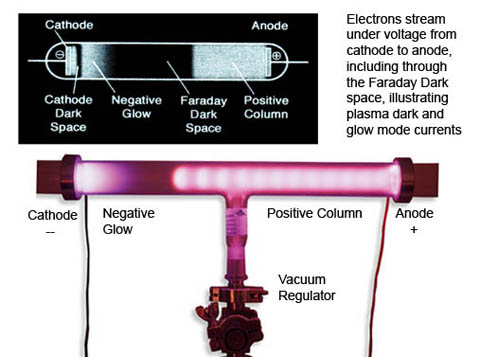
Evacuated (low pressure gas) tube with anode and cathode and high-voltage power source. Image credit: Wiki Creative Commons
6.4 Glow Discharge Tubes
Many descriptions of discharge tubes are available and will not be
repeated here in detail. The salient points for the present purposes are
as follows:
- Within the tube, there are visible bands along the axis wherein the plasma is seen to glow, interspersed with ‘dark’ bands where there is no such glow. The different bands represent two of the three possible modes of operation of plasma when carrying a current.
- The dark bands represent, unsurprisingly, the Dark Current Mode. In these regions the electron velocity is below that necessary to cause visible excitation of the atoms of neutral gas, although ionization will start to occur at higher currents. However, radiation will be given off at wavelengths outside the visible even in the Dark Current Mode and so may be detected by non-optical means.
- The glowing bands represent the Normal Glow Mode. Here, the velocity of the electrons causes ionization to occur. The glow is caused by radiation from the electrons of neutral atoms after they have been excited by collisions with fast free electrons.
- The third possible mode of plasma operation is the Arc Mode, familiar in painfully bright welding applications or lightning, for example.
- Returning to the glow discharge tube, one might expect that the potential difference between the electrodes would cause a uniform electric field along the length of the tube. However, the plasma behaves differently.
- It is found that a Double Layer (DL) develops in the tube which modifies the externally applied electric field between the anode and cathode. The DL forms in such a way that the majority of the potential drop occurs across the DL. Away from the DL region, much of the remainder of the plasma is a glow discharge region known as the positive column. This can extend for a significant part of the length of the discharge tube.
- Within the positive column there are approximately equal numbers of electrons and ions. The plasma here is therefore quasi-neutral. Because most of the potential drop occurs across the DL, only a small but constant voltage gradient, or electric field, exists within the positive column.
- There appear to be analogies between the positive column in a discharge tube and the plasma within the Sun’s heliosphere.
- Another result of the discharge tube
experiments is also relevant to our discussion of plasma behavior and
will be discussed in the next section.
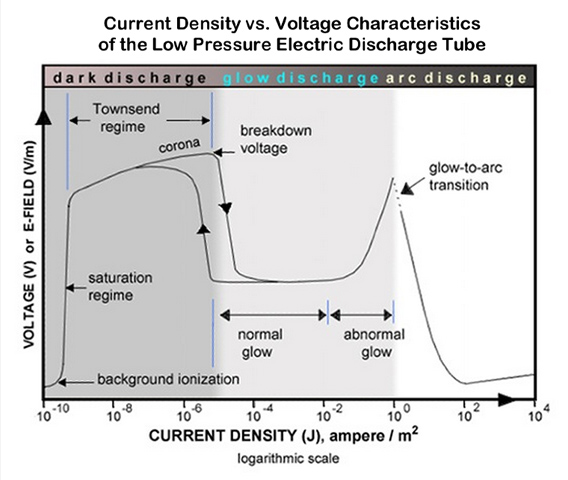
6.5 The Current Density–Voltage Curve
If the current density J (x-axis) is plotted against the voltage V
(y-axis) in a discharge tube (current density is the current divided by
the area of the discharge tube), then it is found that the three
different plasma glow modes correspond to three different sections of a
discontinuous graph, known as the current vs voltage or J-V curve.
In the dark discharge mode,
the J-V curve rises with increasing voltage, although not regularly.
Once the voltage reaches a high enough value, ionization begins and the
current starts to rise very rapidly for very little increase in voltage.
The discharge will then change rapidly into the glow discharge mode.
This is accompanied by a dramatic step change in the voltage. The
voltage drops because, when large numbers of electrons have been
produced by ionization, only a small voltage is needed in order to
generate a large current.
A very significant effect often
occurs in the lower current density part of the glow discharge region.
The voltage actually decreases with increasing current density. In other
words, the plasma finds it more efficient to transmit the current at a
higher current density because the voltage drop is less.
At still higher current densities, the
voltage increases again, meaning that the glow discharge section of the
J-V curve has a minimum at a particular value of current density. This
minimum represents the point of lowest resistance for transmission of
the total current. In cosmic plasmas, this effect may be significant in
causing the formation of current filaments by confining the current
within a particular cross-sectional area.
Similarly, in the extremely bright arc discharge mode,
the voltage once again decreases with increasing current density. If
plasma is forced into the arc mode, it will again tend to filament in
order to reduce the voltage drop.
6.6 Current Filamentation
Filamentation is observed to be a normal
behavior mode for currents in a plasma, as evidenced by the J-V curve
and by physical structures in space itself. A paper by Dr. Anthony
Peratt regarding filamentation can be found here.
In particular, current sheets (which we
will consider later) tend to break up into individual filaments due to
the development of vortices. These vortices are somewhat similar to
those found in fluid flows with adjacent layers of different flow
velocities (Kelvin-Helmholtz instabilities).

A small plasma lamp’s central cathode is surrounded by an excited spherical current sheet which is attracted to the outer glass shell (anode). Where the current extends outward from the surface sheet it forms filamentary structures as it propagates through the low pressure medium within the lamp. Photo credit: Luc Viatour /
www.lucnix.be
Clearly, the conditions inside a current
filament are going to be different to those in the rest of the plasma.
This causes a current-free double layer (CFDL) to form at the boundary
of the filament in the normal way, such that the faster electrons are
confined to the filament by the electric field within the DL.
We can now see that filaments are current-carrying elongated plasma cells with CFDLs at their boundaries.
Evidence of filaments_and_electric_currents in
space is widespread. Filamentary structure is acknowledged by most
astronomers to exist at all levels, from the solar system to galactic
and intergalactic scales. The only area of disagreement between the
Electric Model and the Gravity Model is whether these filaments are
current-carrying structures, naturally following the laws of plasma
electrodynamics, or somehow fluid ‘jets’ thousands of light-years long, gravitationally driven in accordance with computer simulations of the hypothesized gravity forces due to cold dark matter (CDM).
In a fluid, jets tend to dissipate rapidly into low-velocity plumes.

An aircraft’s turbines expel jets of gas, seen here as contrails of ice crystals precipitating some distance aft of the engines, which quickly expand and decelerate to a stop in the upper atmosphere
However, some jets in space, for example
the 4,000 light-years-long jet from the elliptical galaxy M87, appear
to remain in the jet state for enormous distances before dissipating
into a plume. This might indicate that the jets are not fluid jets but
electrical filaments.

The jet from galaxy M87. Galaxy is the bright knot, upper left, in visible light (reddish); the jet extends down and to the right, seen here in UV light (white and blue). Image credit: NASA/ Hubble
A significant paper titled, “Measurement of the Current in a Kpc-Scaled Jet” was published in 2011 in arXiv by Kronberg, Lovelace, et al, based on their investigations of a jet emanating from radio galaxy 3C303
If we assume they are electric
filaments, then we need to know what theory and experiment might tell us
about how electric filaments keep their shape over astronomical
distances. This is discussed next.
6.7 Current Pinches
Any current I flowing in a conductor or filament will cause a magnetic field B
around it. The lines of equal magnetic force will be in the form of
rings around the axis of the current. The magnetic force will decrease
with radial distance from the axis.
From consideration of the Lorentz Force, it can be shown that the interaction of the current I with its own magnetic field B will cause a pressure radially inward on the current filament, written as I × B
(that is, “I cross B” in vector terminology). This is called a ‘pinch’
or ‘z-pinch’ (when defining the current flow as parallel with the ‘z’
co-ordinate’s direction).
In a metal conductor, the I × B
pressure is resisted by the atomic ion lattice. In a plasma current,
the pressure can be balanced by the pressure of the plasma inside the
filament. This results in a steady state where the current can flow
axially across its own azimuthal or circling magnetic field. The
balancing equation is known as the Bennett Pinch equation.
Lab demonstrations can use the pinch
effect to crush aluminum cans by applying a strong magnetic field very
quickly. The can is crushed before the pressure in the can is able to
build up sufficiently to resist the pinch force. Magnetic field forces
in lightning can create an inward pinch that will crush a solid copper
grounding rod.

Left: The field generated by a fast 2 kj discharge through 3-turn heavy wire crushed this can. Right: Nature’s lightning z-pinch deformed this metal rod. Images credit: Wiki Creative Commons
6.8 Field-Aligned Currents
In space, the neutral gas pressure is usually negligible, and so the balance between the I × B force and the pressure force cannot occur. The only way the situation can be resolved is for the I × B
force to disappear. This implies that I and B (current direction and
magnetic field direction) are parallel and, by vector algebra, the cross
product is zero.
If other magnetic fields are present, as they are known to be through much of cosmic space, then the I × B force must be calculated using the total magnetic field, that is, by adding the current’s own B to the general B, added using vector algebra.
Thus in a space plasma, the current I and the total magnetic field B realign so as to be parallel. In other words, the current follows the magnetic field: it is a ‘field-aligned’ current.
Even if there is no external magnetic
field, any small elements of current flowing in a plasma will tend to
accumulate naturally into larger currents which generate their own
magnetic fields and so preserve the filament of current.
What happens is that electrons nearer
the centre of the filament flow in almost straight lines and generate an
azimuthal magnetic field around them. Electrons further from the centre
are influenced by this azimuthal component of the magnetic field and
move in a more helical path aligned with the main current direction.
This helical motion creates the straighter magnetic field lines near the
axis, as shown in the following diagram. The nearer the centre of the
filament, the straighter are the magnetic field lines and the paths of
the electrons.

Electron flows in a field-aligned current at varying distances from the center of the current filament. Image credit: Wiki Commons
Any individual electron in the current
is thus flowing along the magnetic field direction in its own vicinity,
but collectively the filament is preserved even without an external
magnetic field. This means that very large currents can be assembled out
of small current elements and transmitted over huge distances.
Another way of looking at this is to
consider the electrical resistance of the plasma. Current flowing across
the magnetic field direction will experience more resistance than
current flowing along the magnetic field direction because of the U × B
term in the Lorentz Force Law. Effectively, the parallel resistance is
less than the perpendicular resistance, so the current tends to flow in
alignment with the magnetic field.
6.9 Self-Constriction of Currents
Detailed mathematical analysis shows that I and B interact in such a way that both I and B tend to spiral parallel to each other around an axis aligned with the external B. The net effect is that I and B both follow a helical path aligned with the direction of the external B field.
It is also found that the interaction of the axial and azimuthal (ring) components of the helical I and B cause both I and B to be largely confined to a cylinder of definite radius centered on the axis.
To summarize, the absence of significant pressure in space plasmas causes currents to flow in cylindrical filaments aligned with the general magnetic field direction.
Within the cylindrical filament, both the current and the magnetic
field will spiral around the axis of the cylinder whilst remaining
parallel to each other.
Note that if for any reason the parallel alignment between I and the total B is disturbed, then an I × B
force will arise and cause either radial compression or radial
expansion, depending on which of the two components is more axial. Thus
pinching of a filament could occur because, for example, of changes in
the fields through which the current filament was flowing.
6.10 Stability of Current Filaments
Another significant factor emerges from
the mathematical analysis. The force-free or field-aligned arrangement
is a minimum energy state for the current to flow in. This means that
the field-aligned arrangement is inherently stable. Unless disturbed by
external factors, currents will tend to remain aligned with the magnetic
field.
We can now see how field-aligned
currents can persist over vast distances. Field-aligned currents are
therefore a much more likely explanation of the collimated
(parallel-flow) ‘jets’ seen to be extending for hundreds to thousands of
light-years than is the Gravity Model explanation based on conventional
fluid flows (refer to 6.6 above). The UK radio observatory at Jodrell Bank has collected a wide variety of images of galactic jets seen in radio wavelengths, in their Atlas of DRAGNs (Double Radiosource Associated with Galactic Nuclei) One such image is depicted below, showing a typical double jet:
The confinement of field-aligned filamentary currents to definite cylinders of current by electromagnetic forces is also consistent with the falling characteristic of the J-V curve seen in laboratory experiments in discharge tubes. If the plasma is in Glow Mode, which in space plasmas may mean a glow in wavelengths outside the visible range, then the radius of the current cylinder will be determined by a combination of the effects of the electric and magnetic fields and the shape of the current density-Voltage curve. Read more about the filamentation process in dense cosmic z-pinches in this_paper by Russian physicists A.B Kukushkin and V.A. Rantsev-Kartinov of the Kurchatov Institute, Moscow.
6.11 Condensation of Matter
A further effect related to the I × B force can also be determined by analysis. Suppose that the current I is caused by an electric field E. Now consider the force arising from the interaction of E and B. Remember that I tends to become aligned with the total B due to the forces on the current itself. Then the E causing the current will not be entirely aligned with the total B,
which is the vector sum of the external magnetic field through which
the current flows and the azimuthal magnetic field generated by the
current itself.
As with the I × B force, there is also an E × B force, whenever E is not parallel to B. This E × B
force acts on charged particles in the current cylinder and causes both
ions and electrons to move towards the centre of a filament. Plasmas
often contain a high proportion of charged dust grains, which will also
be drawn into the filament. Viscous drag between the charged particles
and neutral atoms will tend to draw the neutral atoms towards the
filament as well.
Therefore, current filaments in space
will tend accumulate matter in them as a result of the misalignment of
the electric field causing the current and the total magnetic field.
Remembering that pinches can occur if any misalignment of I and B occurs, any matter that has been drawn into the filament will also be compressed if a misalignment of I and B
occurs. If the pinch force is large enough, it can fragment the
filament into discrete spherical or toroidal plasmoids along the axis of
the current. Any matter in the pinch zone would then become compressed
into the same form.
Because the electromechanical forces are
vastly stronger than gravity, this mechanism offers a means by which
diffuse matter can be accumulated and compressed in a much more
efficient way than gravitational compression of diffuse clouds of fine
dust particles.
[ this provides a protocol for matter accumulation that satisfies the needs of star making - arclein ]
Of course, once the matter has been sufficiently compressed and if it is neutralized by recombination of ions and electrons, then the electromagnetic forces may be reduced to the point that gravity becomes significant and continues the compression started by the electromagnetic forces.
Of course, once the matter has been sufficiently compressed and if it is neutralized by recombination of ions and electrons, then the electromagnetic forces may be reduced to the point that gravity becomes significant and continues the compression started by the electromagnetic forces.
6.12 Marklund Convection
In the case of a cylindrical current, the E × B
force is radially inwards and results in the self-constriction of a
current filament, as we have seen. This results in an increase in the
particle density near the axis of the current. Two things can then
happen.
- The first is that radiative cooling from the regions of increased density can result in a temperature decrease nearer the center, contrary to the increase one might intuitively expect from increasing the density.
- The second is that recombination of ions and electrons starts to occur.
Every chemical element has a particular energy level, known as its ionization energy,
at which it will either ionize or recombine. This is analogous to the
boiling point of a liquid such as water: at a particular temperature,
the phase or state of the matter will change from one state to another.
If the kinetic energy of motion is equated with the ionization energy, then a characteristic velocity, known as the Critical Ionization Velocity (CIV),
can be derived for each element. Because temperature is a measure of
thermal energy, CIV can be related to temperature. The CIV values of
elements commonly found in space are not distributed randomly but are
grouped into four distinct bands around certain velocity values. Within
each band, all the elements in that band have similar CIVs to each
other.
In the vicinity of a field-aligned current, the E × B
force causes a radial drift of ions and electrons towards the cooler
central axis. Because of their differing CIVs, different ions will
recombine at different radii as they move towards the centre and enter
progressively cooler regions.
This process is known as Marklund Convection after the Swedish physicist who discovered it, Göran Marklund.
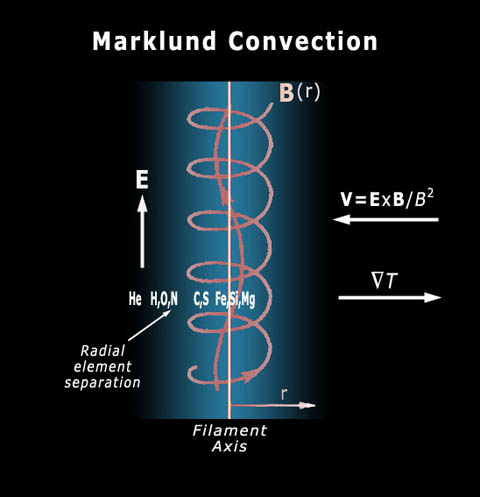
Marklund convection and sorting in a magnetically pinched current. Image courtesy of Wal Thornhill, www.holoscience.com
The net result is that Marklund
Convection sorts any elements present in the locality into different
groups according to their ionization potentials. The groups of elements
are arranged in cylindrical shells at different radii within a
cylindrical field-aligned current.
As hydrogen has a high CIV compared to the other elements, it will recombine first, in a cylindrical shell of larger radius than the shells of the other elements.
This type of electrical sorting may be
responsible for some of the non-random distribution of elements that we
observe in the cosmos. In particular, it may explain the preponderance
of neutral hydrogen in thread-like structures throughout the galaxy that
have been detected by radio telescopes.

Could this Eagle Nebula image by the Hubble Space Telescope be an illustration of a cosmic magnetic pinch and resultant dusty plasma surrounded by a hydrogen-helium environment?
End of Chapter 6

No comments:
Post a Comment